I started using math centers earlier this year in an attempt to solve the problem of how to address a broad range of skills and diversity of student learning needs in the typical math classroom. Although centers offers a differentiated approach that frees the teacher up to work with individuals and small groups, I have found that it requires considerable set up and lots of learning skills and work habits "training" for the students. This year I also noticed that when I was only using a centers-based approach, I was missing teaching more "whole class", 3-part lessons.
This morning, as we had another classroom visitor to observe centers in action as part of our current (Transformational Geometry) unit, I had the opportunity to consider my problem of practice and theory of action yet again.
Unlike our recent Fractions unit, which was almost exclusively taught through a centers-based approach, Transformational Geometry is being taught in room 312 through a combination of 3-part lessons, computer lab sessions (for the Grade 7s with whom I have time in the lab), and some centers.
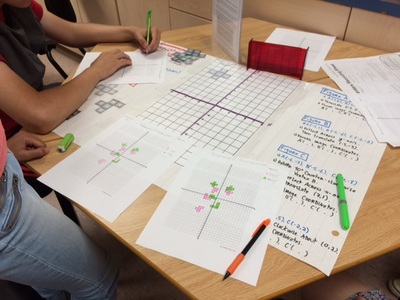
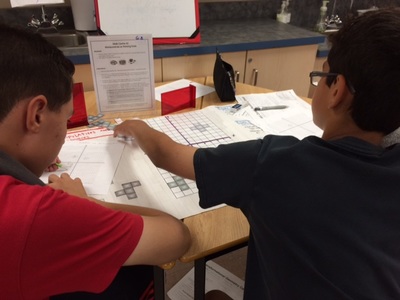
Today was the first of two "Centers Days" for Transformational Geometry.
| geometry_centers_recording_page.docx |
An idea gleaned from today's visit revolves around Center 7 (my "math games" center): We noticed that several students played Blokus, Katamino and Battleship without really making the connection I hoped they would between the concepts they were using to try and win, and the success criteria posted. For future units, a change I could make would be to include with this center a short list of game-specific questions that students must respond to while at this center. (They could also be used by teachers when working with students at this center.) Questions could be scaffolded to lead students to certain conclusions, and a copy of the unit vocabulary made available at the center to foster improved oral and written math communication about the game.
Using Centers Days as part -- but not all -- of my math units is helping me to better appreciate their intrinsic value and refine and refocus their value as a learning tool for students involved in a student centered, balanced math program.




 RSS Feed
RSS Feed